TIDE-GENERATING FORCES
Dynamics of Earth-Moon System.-The moon attracts every particle
of the earth and ocean. By the law of gravitation the force acting on any
particle is directed toward the moon's centre, and is jointly proportional
to the masses of the particle and of the moon, and inversely proportional
to the square of the distance between the particle and the moon's centre.
If one can imagine the earth and ocean subdivided into a number of small
particles of equal mass, then the average, both as to direction and intensity,
of the forces acting on these particles. is equal to the force acting on
that particle which is at the earth's centre. If every particle of the
earth and ocean were being urged by equal and parallel forces there would
be no cause for relative motion between the ocean and the earth. Hence
it is the departure of the force acting on any particle from the average
which constitutes the tide-generating force. Now it is obvious that on
the side of the earth toward the moon the departure from the average is
a small force directed toward the moon; and on I the side of the earth
away from the moon the departure is a small force directed away from the
moon. All around the sides of the earth along a great circle perpendicular
to the line joining the moon and earth the departure is a force directed
inward toward the earth's centre. Thus it can be seen that the tidal forces
tend to pull the water toward and away from the moon, and to depress the
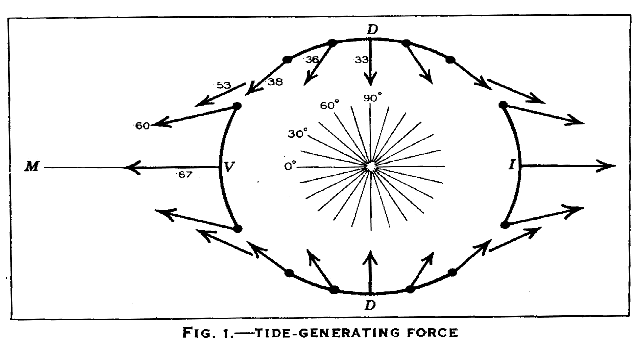
water at right angles to that direction. In the figure below this distribution
of forces is illustrated graphically. The relative magnitudes of the forces
are given by the numbers on the figure, M being in the direction of the
moon. The separate attractions of the moon at the earth's centre and at
a point on the earth's surface are each inversely proportional to the square
of the moon's distance, so that the difference between the two which gives
the tide-generating force, is approximately inversely proportional to the
cube of the moon's distance.
The vertical component of the tide-generating force coincides in direction with the gravitational force of the earth itself, and thus acts as a slight modification of weight. This component does not tend to alter the position of equilibrium which the water would take up in the absence of any disturbance from an extraterrestrial body. The effective tide-generating forces, therefore, are the horizontal components of those indicated in the figure.
Equilibrium Form of the Tide.-For many purposes it is convenient to specify the distribution of the tide-generating forces at any instant by reference to a fictitious tide. If a completely rigid earth were completely covered by a layer of ocean, and if the tide-generating forces were to remain constant, there would be an invariable elevation of water at each point of the ocean surface and no tidal currents. If the distribution of forces were the same as that of the actual tide-generating forces at any instant, then the consequential elevation of water might be used as a specification of these forces. This distribution of fictitious tidal elevation is known as the equilibrium form of the tide. It is a real and accurate specification of the actual tide-generating forces throughout the earth's body as well as on its surface. When the equilibrium form is stated for every instant there is a complete specification of these forces, and henceforth the terms equilibrium tide and tidegenerating force will be used interchangeably,
In this equilibrium form the inclination, to the horizontal, of the surface of the water would be always such that the consequential pressure gradient would everywhere balance the tide-generating force. - It is therefore clear that the surface of the water would slope upward from DD toward the points V and I, the water being raised by a maximum amount at V and I and depressed by a maximum amount along the great circle through DD. The surface of the water would be nearly that of an ellipsoid of revolution. The volume of this ellipsoid would be the same as that enclosed by the surface of the undisturbed ocean. Owing to the motion of the moon relative to the earth, the tidal ellipsoid moves over the earth so that V is always directly under the moon and I always directly opposite, while owing to the varying distance of the moon the equilibrium ellipsoid, changes slightly in shape.
The tide-generating forces attributable to the sun's gravitation may be similarly specified. The sun's mass is nearly 27,000,000 times the moon's mass and the sun's distance is about 390 times the moon's distance from the earth. Consequently the sun's tidegenerating forces are to those of the moon in the ratio Of 27,000,000 to 3903; i.e., 0.460 or 1/2.17 . This means that at corresponding points of the two ellipsoids representing the lunar and solar equilibrium forms the tidal elevations on the average will be in the ratio of 2.17 to 1.
Harmonic Constituents-The study of tides on the earth has been greatly facilitated by the fact that the tidal motions, whether in the atmosphere, hydrosphere or lithosphere, are to a high degree of precision linear with respect to the tide-generating forces which cause them. This will be demonstrated later in the case of the dynamics of the ocean tide.
The significance of this linearity is that the tide-generating force can be considered as the sum of a number of constituents, the actual tide being the sum of the individual effects that each constituent would produce separately in the absence of all the others. As an example, the lunar and solar tides can be considered separately. The tide which would be produced under the combined action of moon and sun would be almost exactly what one would obtain by adding the separate contributions of moon and sun. In other words, there is little interaction between the lunar and solar tides.
The tide-generating forces can be expressed quite precisely by a finite series of harmonic terms, and in practice it has been found that about 30 terms generally give an adequate approximation. Furthermore, linear systems have the important property that their response is harmonic when they are acted upon by a force varying harmonically with time, the response having the same frequency as the force. The above two facts form the basis of the harmonic development of the tide, which has been in use since the time of Pierre S. Laplace.
Each term of the series representing the elevation
of the equilibrium tide at some particular point on the earth's surface
will be of the form 
, where t is time, H is the amplitude, n is the frequency
or speed (commonly expressed in units of degrees per hour), and 
is the epoch. For any given constituent the speed is constant, while the
amplitude and epoch are constant in time but may depend on geographical
location. The harmonic terms representing actual tides will be of the same
form. In the case of the ocean tides H may represent the amplitude
of the elevation of the water surface relative to the ground; for bodily
tides H may represent the amplitude of a component of particle displacement;
and for atmospheric tides H may represent the amplitude of a pressure
fluctuation.
Let us now consider the effect of only one tide-generating
body, such as the moon. As demonstrated in the previous section, the tide-generating
forces of the moon can be specified completely by the shape and geographic
orientation of the tidal ellipsoid, whose major axis is oriented along
the line joining the centres of earth and moon. Now for the moment, let
us consider what would happen if the moon remained at a constant distance
from the earth and revolved about the earth in the equatorial plane with
constant speed. An observer located at any given geographical position
would experience two maxima and two minima of the equilibrium tide each
lunar day. The amplitude would be a maximum at the equator and would decrease
with increasing northern or southern latitude to a value of zero at the
poles. The rise and fall of the equilibrium tide would be approximately
harmonic with speed 
where 
denotes
the angular speed of the earth's rotation relative to the stars and 
the mean speed of revolution of the moon about the earth, and the equilibrium
tide would be represented very closely by a single harmonic constituent.
Since the moon's speed of revolution is small compared to the earth's speed
of rotation, the period of this constituent is approximately semidiurnal.
The moon's varying declination (angular distance from equatorial plane),
varying apparent angular speed of revolution about the earth and varying
distance from the earth cause many modifications in this simplified example.
If the moon revolved about the earth with constant angular speed at
some constant declination not equal to zero, one of the two poles of the
equilibrium tidal ellipsoid would be always in the northern hemisphere,
and the other in the southern hemisphere. The two equilibrium high waters
that a point on earth not on the equator would experience* as the earth
rotates would therefore not be equal, and the introduction of a new constituent
of speed 
,
approximately diurnal, would be required. This effect is known as the diurnal
inequality. The variable declination of the moon results from the moon's
motion in an orbit whose plane intersects the earth's equatorial plane
at an angle I. The lunar declination thus passes through a monthly
cycle but since both north and south declinations have essentially the
same effect, i.e., that of decreasing the semidiurnal. and increasing the
diurnal contribution, the two constituents suffer a fortnightly modulation.
Strictly
speaking, such constituents cannot be considered harmonic, as there is
implied in this term the invariability of amplitude, speed and epoch. These
constituents can, however, be expressed precisely as the sum of a number
of strictly harmonic terms, and more will be said about this point later.
The distance from earth to moon varies throughout a monthly cycle giving rise to a monthly modulation in the amplitude of the equilibrium tide. The maxima occur when the moon is at perigee, the point on the moon's orbit which is closest to the earth; the minima occur at apogee, when the moon is farthest from the earth. The time between successive lunar passages through perigee or apogee is the so-called anomalistic month, whose average length is 27.55 (mean solar) days. The speed of revolution of the moon about the earth varies inversely with the moon's distance according to Kepler's laws (see ORBIT). This causes the epoch of the equilibrium tide to vary according to the moon's position in its orbit, resulting in an apparent increase of period at apogee as compared to perigee. This monthly modulation of both amplitude and epoch of the equilibrium tide can again be expressed by a sum of purely harmonic constituents.
There are many other irregularities in the moon's motion, all of which can be expressed by the addition of more harmonic terms insofar as their effect on the equilibrium tide is concerned. The plane of the lunar orbit does not remain with constant orientation in space. Its movement is best described by the motion of the moon's nodes, which are the two points on the lunar orbit which lie on the plane of the ecliptic. Relative to the stars these nodes describe a westward motion known as the regression of the nodes, completing a cycle in about 19 years. Also, the major axis of the lunar orbit revolves eastward relative to the stars, completing a cycle in about nine years.
The solar effects are in every way analogous to the corresponding lunar effects.
Species.-In the above section it was seen that many cases arise
where a constituent having a slow periodic variation of amplitude or epoch
can be represented by the sum of two or more purely harmonic constituents.
The speeds of these purely harmonic constituents will belong to the series, 
,
where n is the speed of the original constituent and 
is the speed corresponding to the fundamental period of variation of its
amplitude or epoch. If
is small as compared to n, the speeds of the purely harmonic constituents
will cluster closely about the speed of the original constituent. In the
case of the actual tide, most of the constituents are approximately semidiurnal,
diurnal, or of a longer (fortnightly or longer) period of variation, and
their amplitudes and epochs pass through a much longer period of variation
than that of the actual constituent. As a result, the periods of purely
harmonic constituents representing the equilibrium tide are clustered about
12 hr., 24 hr. and much longer periods, offering a logical basis for classification
of the tidal constituents.
The scheme of classification shown in Table I was developed by George Darwin in 1882 and has been adopted by most of the world's tidal authorities. Within any given species the coefficient is proportional to the equilibrium amplitude, and this proportionality holds roughly between constituents belonging to different species. The subscript on the symbol designating the constituent refers to the species to which it belongs. The long-period constituents have no subscript, the diurnal species have the subscript "one," the semidiurnal species the subscript "two." In addition to the constituents tabulated in Table I, there are terdiurnal, 4-diurnal, etc., constituents in the equilibrium tide, but these are of much lower amplitude and are not of much importance on earth.
TABLE I--Tidal Constituents
|
|
||||
|
|
|
|
|
|
|
|
|
 |
|
|
|
|
|
 |
|
|
|
|
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
|
|
 |
|
|
|
|
|
 |
|
|
|
|
||||
|
|
|
 |
|
|
|
|
|
 |
|
|
|
|
|
 |
|
|
|
|
|
 |
|
|
The earth's speed of rotation
is the largest of all the parameters contributing to the speeds of the
tidal constituents, and its numerical multiplier in the expression for
the speed determines to which species a given constituent belongs. The
other quantities in Table I which determine the speeds of the constituents
are the mean motion of the moon ,
the mean motion of the sun 
,
the speed of revolution of the moon's nodes N, and the mean motion
of the lunar perigee w. These quantities have a smaller effect and
merely determine the period of the constituent within its species. It can
therefore be said that the fundamental reason that the tidal constituents
clump conveniently into species is that the day is much shorter than either
the month or the year.
The equilibrium constituents of any given species all have the same geographical form. The long-period constituents are characterized by a standing wave symmetrical about the earth's axis. The polar regions oscillate in phase with each other and are 180° out of phase with the equilibrium tide in low latitudes. The two nodal lines lie along latitudes 35.26° north and south. The diurnal constituents have the form of a progressive wave rotating relative to the earth about its axis from east to west. At any given instant there are two maxima at opposite extremes of the earth and two minima similarly situated, all lying at latitudes 45° north and south on a great circle passing through the earth's poles. The amplitude of the diurnal equilibrium tide vanishes at equator and poles. The semidiurnal constituents also have the form of a progressive wave rotating about the earth from east to west. There are two maxima on the equator at opposite extremes of the earth and two minima also on the equator 90° from the maxima. The amplitude of the semidiurnal equilibrium tide is maximum at the equator and dies off with increasing latitude, vanishing at the poles.
Description of the Constituents.--Let us consider first the semidiurnal constituents. As can be seen from the value of their coefficients tabulated in Table I,
The constituents Mf and Ssa result from the variable declination of the tide-generating body and are at a maximum when this body is at zero declination. The lunar fortnightly constituent Mf thus reflects the variable declination of the moon, and the solar semiannual constituent Ssa similarly reflects the variable declination of the sun. In addition there is a small 19-year constituent which arises from the 19-year cycle in the regression of the moon's nodes, as well as many other minor long-period constituents.
In Darwin's harmonic development the tide-generating forces (the equilibrium tide) are not analyzed into strictly harmonic constituents; that is, the amplitudes and epochs of Darwin's constituents are not truly constant. They exhibit the 19-year cycle of the regression of the moon's nodes. However, during any one year the amplitudes and epochs vary so slightly that the constituents can be considered as true harmonic terms without incurring appreciable error. The nodal variation in amplitude is accounted for by taking the constituent coefficients c (those values tabulated in Table I) to be constant in time while denoting the true amplitude of the equilibrium constituents by the quantity fc where f is called the node factor and is the quantity which varies. The mean value of f is taken to be unity, and its values have been tabulated for each year for all important constituents. The value of f changes so slowly that it is adequate to take its mean value during any given year in place of its true value at any instant during that year.
In 1921 Doodson developed the equilibrium tide into a series of harmonic constituents whose amplitudes and epochs do not exhibit this 19-year cycle, and therefore do not need the artifice of node factors. For this reason it may be said that Doodson's development is more nearly perfectly harmonic than is Darwin's. Even though Doodson's development does not attempt to take into account such long-term variations as secular changes in the distance between earth, moon and sun, and geologic changes in sea level, it is doubtful that a more elaborate development will ever be needed for tidal work.