Math 2A  Vector Calculus  The Wave Equation  Fall ‘07

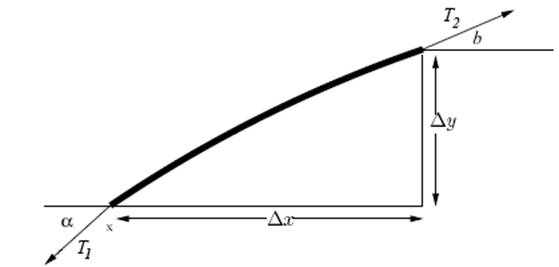
Consider an elastic string which is stretched to length L and then fixed at the end points.  Suppose that the string is plucked at t = 0 and allowed to vibrate. The problem is to determine the vibrations of the string, that is, to find its deflection u(x,t) at any point x and at any time  t > 0.  When deriving a differential equation to model a physical problem, we make simplifying assumptions so the resulting equation doesn’t get too complicated. In this case we assume:

                     i.      The mass of the string per unit length is constant (''homogeneous string'').

                   ii.      The string is perfectly elastic and does not offer any resistance to bending.

                  iii.       The tension caused by stretching the string before fixing it at the end points is so large that the action of the gravitational force on the string can be neglected.

                 iv.      The motion of the string is a small transverse vibration in a vertical plane, that is, each particle of the string moves strictly vertically, and the deflection and the slope at any point of the string are small in absolute value.

To obtain the differential equation, consider the forces acting on a small portion of the string, say from x to x + Δx..  Since the string does not offer resistance to bending, the tension is tangential to the curve of the string at each point.  Let T₁ and T₂ be the tensions at the end points P and Q of the small portion of string under consideration.  Since there is no motion in the horizontal direction, the horizontal components of the tension must be constant.  

Using the notation of the figure above, we have

                                             (1)    

In the vertical direction we have two forces, namely the vertical components  and  of  T₁ and T₂ ; here the negative sign occurs because that component of P is directed downward.

By Newton's second law, the resultant of those two forces is equal to the mass ρΔx of the small portion of string times the acceleration , evaluated at some point between x and x + Δx.  That is,

                                        (2)    

Combining equations (1) and (2) above, we have

                                         (3)      

Now  and  are the slopes of the tangents to the string’s curve at P and Q.  That is,

Dividing both sides of (3) by Δx and substituting from above,

Taking the limit as Δx → 0 we obtain the wave equation  where .

Now since the string is fixed at the endpoints, we impose boundary conditions

We can describe the initial displacement as some function

Problems:

1.      Show that if we assume  satisfies the wave equation, then .

2.      Explain  means that both the left side and the right side must be constant so that  and .

3.      Show that  and  where  yield solutions  satisfying the boundary conditions.

4.       and so  are the coefficients that yields a solution satisfying the initial conditions