Math 1A – Chapter 4.1 – 4.4 Test Solutions – Fall ’04
|
1.
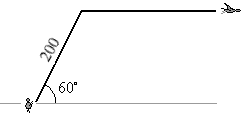
A falcon flies up from its trainer at an angle of 60◦
until it has flown 200 ft. It then
levels off and continues to fly away.
If the speed of the bird is 132 ft/sec, how fast is the distance between
the bird and the falconer increasing after 6 seconds?
|
|
SOLN: Let x = the horizontal leg of the falcon’s
flight path and let D = the distance
from the falconer to the falcon. Then by
the law of cosines, . Differentiating with respect to x, and substituting we have . After 6 seconds, the falcon has flown a total
distance of 132*6 = 792 feet, so x =
592. That means that .
Thus feet per second.
|
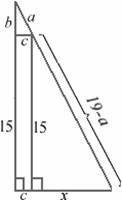
2. A ladder 19 ft long leans against a 15 foot wall so the top of the ladder juts over the top of the wall. If the base of the ladder slides away from the wall at the rate of 3 ft/sec, find the rate at which the height of the top end of the ladder is decreasing when 2 feet of ladder projects over the wall. |
|
|
SOLN: Drop a plumb line down from the top of the ladder down to the ground and call it 15+b. where b is the vertical leg of the right triangle whose hypotenuse is the tip of the ladder extending above the wall. We seek . Let a and c be the other two sides of the triangle, as shown at right. Let x = the length from the base of the ladder to the base of the wall so that . By Pythagorus, . Differentiating, so that when a=2, . Thus we |
|
find . From similar triangles we have . Differentiating, and when a=2,
so that ft/sec.
3. Find a formula for a function that
a.
… is continuous on and has an absolute maximum, but no absolute
minimum.
SOLN: There are a great variety of
possible solutions here. Perhaps the simplest is a line segment through the
origin with slope –1: y = –x which achieves an absolute maximum of
1 at x = –1 but never attains a
minimum since all points slightly above
y = –2 are included but –2 itself.
b.
…is discontinuous on but achieves both an absolute maximum and an
absolute minimum.
SOLN: Again, there are too many
different types of solutions to even catalogue the types here. All we need is one. ,
the greatest integer less than x/3,
will do. There is a discontinuity where x = 0. The absolute minimum is –1, which is attained
for all x in [–1,0) and the absolute
maximum is 0, which is attained for all x
in [0,2].
4. Consider .
a.
Use a graph to estimate where the absolute maximum and
minimum occur.
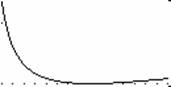

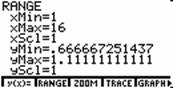
On the TI-85 enter the formula as y1=1/√x+√x/9
and do a zoomfit on the interval 1≤x≤16.
The resulting graph (first of the screen shots above) appears to have a
critical point (horizontal tangent line) about midway between x=1 and x =16. Using the FMIN feature on the
b.
Use calculus to find the exact values of these
extremes.
SOLN: The maximums that occurs at the
left endpoint is at (1, 10/9). The
minimum occurs where ,
so the minimum occurs at x=9 where .
5. Consider
a.
Find the intervals on which x is increasing or decreasing.
SOLN: only if ,
so x is decreasing on and increasing outside of that interval; that
increasing on .
b.
Find the local maximum and minimum values of x.
SOLN: The local max is where x stops increasing and starts
decreasing: at . The local min is where x stops decreasing and starts increasing: (0,0).
c.
Find the intervals of concavity and the inflection
point.
SOLN: changes sign at t = –2/3, so the inflection point is at .
6.
Find values of a
and b so that has an absolute maximum at .
SOLN: If the curve is to pass through
the required maximum then . ,
so at x = ½, ,
so a = 4 also.
7. Consider the curve defined parametrically by where .
a.
For what values of t
does the curve have vertical tangent lines?
SOLN: There is a vertical tangent line
where x’(t) = 0, that is
b.
For what values of t
does the curve have horizontal tangent lines?
SOLN: There is a horizontal tangent line
where y’(t) = 9, that is